Macro-economy - REMIND-MAgPIE: Difference between revisions

From IAMC-Documentation
Jump to navigation Jump to search
No edit summary
m (Text replacement - "RICE" to "RICE50+")
 
(25 intermediate revisions by 5 users not shown)
Line 1: Line 1:
{{ModelDocumentationTemplate
{{ModelDocumentationTemplate
|IsDocumentationOf=REMIND
|IsEmpty=No
|IsDocumentationOf=REMIND-MAgPIE
|DocumentationCategory=Macro-economy
|DocumentationCategory=Macro-economy
}}
}}
'''Note:''' This pages describes the REMIND 1.7 model. It will be updated shortly to describe the most recent version of REMIND-MAgPIE.
<br>
<br>
==Objective function==
==Objective function==


REMIND models each region r as a representative household with a utility function Ur that depends upon per-capita consumption
REMIND-MAgPIE models each region r as a representative household with a utility function Ur that depends upon per-capita consumption


[[File:REMIND_equation_3.2.1_1.JPG]]
<figure id="fig:REMIND-MAgPIE_3.2.1 1.">
[[File:REMIND-MAgPIE equation 3.2.1 1.JPG]]
</figure>


where Crt is the consumption of region r at time t, and Prt is the population in region r at time t. The calculation of utility is subject to discounting; 3% is assumed for the pure rate of time preference r. The logarithmic relationship between per-capita consumption and regional utility implies an elasticity of marginal consumption of 1. Thus, in line with the Keynes-Ramsey rule, REMIND yields an endogenous interest rate in real terms of 5-6% for an economic growth rate of 2-3%. This is in line with the interest rates typically observed on capital markets.
where C(r,t) is the consumption of region r at time t, and P(r,t) is the population in region r at time t. The calculation of utility is subject to discounting; 3% is assumed for the pure rate of time preference rho. The logarithmic relationship between per-capita consumption and regional utility implies an elasticity of marginal consumption of 1. Thus, in line with the Keynes-Ramsey rule, REMIND-MAgPIE yields an endogenous interest rate in real terms of 5–6% for an economic growth rate of 2–3%. This is in line with the interest rates typically observed on capital markets.  


In the Negishi approach, which computes a cooperative solution, the objective of the Joint Maximization Problem is given as the weighted sum of regional utilities that is maximized subject to all other constraints.
REMIND-MAgPIE can compute maximum regional utility (welfare) by two different solution concepts – the Negishi approach and the Nash approach <ref>Leimbach M, Schultes A, Baumstark L, et al (2016) Solution algorithms of large‐scale Integrated Assessment models on climate change. Annals of Operations Research, doi:10.1007/s10479-016-2340-z</ref>. In the Negishi approach, which computes a cooperative solution, the objective of the Joint Maximization Problem is the weighted sum of regional utilities, maximized subject to all other constraints:


[[File:REMIND equation 3.2.1 2.JPG]]
<figure id="fig:REMIND-MAgPIE_3.2.1 2.">
[[File:REMIND-MAgPIE equation 3.2.1 2.JPG]]
</figure>


An iterative algorithm adjusts the weights so as to equalize the intertemporal balance of payments of each region over the entire time horizon. This convergence criterion ensures that the Pareto-optimal solution of the model corresponds with the market equilibrium in the absence of non-internalized externalities. The algorithm is an inter-temporal extension of the original Negishi approach (Negishi 1972); see also (Manne and Rutherford 1994) for a discussion of the extension). Other models such as MERGE (Manne et al. 1995) and RICE (Nordhaus and Yang 1996) use this algorithm in a similar way.
An iterative algorithm adjusts the weights so as to equalize the intertemporal balance of payments of each region over the entire time horizon. This convergence criterion ensures that the Pareto-optimal solution of the model corresponds with the market equilibrium in the absence of non-internalized externalities. The algorithm is an inter-temporal extension of the original Negishi approach <ref>Negishi T (1972) General equilibrium theory and international trade. North-Holland Publishing Company Amsterdam, London</ref>; see also <ref>Manne AS, Rutherford TF (1994) International Trade in Oil, Gas and Carbon Emission Rights: An Intertemporal General Equilibrium Model. The Energy Journal Volume15:57–76</ref> for a discussion of the extension. Other models such as MERGE <ref>Manne A, Mendelsohn R, Richels R (1995) MERGE: A model for evaluating regional and global effects of GHG reduction policies. Energy Policy 23:17–34. doi: 10.1016/0301-4215(95)90763-W</ref> and RICE50+ <ref>Nordhaus WD, Yang Z (1996) A Regional Dynamic General-Equilibrium Model of Alternative Climate-Change Strategies. The American Economic Review 86:741–765</ref> use this algorithm in a similar way.


The Nash solution concept, by contrast, arrives at the Pareto solution not by Joint Maximization, but by maximizing the regional welfare subject to regional constraints and international prices that are taken as exogenous dates by each region. The intertemporal balance of payments of each region is equal to zero and is one particular constraint that is imposed on each region. The equilibrium solution is found by iteratively adjusting the international prices until global demand and supply are balanced on each market. The choice of the solution concept is also important for the representation of trade, as discussed in Section Trade.
The Nash solution concept, by contrast, arrives at the Pareto solution not by Joint Maximization, but by maximizing the regional welfare subject to regional constraints and international prices that are taken as exogenous data for each region. The intertemporal balance of payments of each region has to equal zero and is one particular constraint imposed on each region. The equilibrium solution is found by iteratively adjusting the international prices until global demand and supply are balanced on each market. The choice of the solution concept is also important for the representation of trade, as discussed in Section the section on Trade.  


In contrast to the Negishi approach, which solves for a co-operative Pareto solution, the Nash approach solves for a non-cooperative Pareto solution. The cooperative solution internalizes interregional spillovers between regions by optimizing the global welfare by using Joint Maximization. The non-cooperative solution only considers spillovers, but they are not internalized. The relevant externalities are the technology learning effects in the energy sector.
In contrast to the Negishi approach, which solves for a co-operative Pareto solution, the Nash approach solves for a non-cooperative Pareto solution. The cooperative solution internalizes interregional spillovers between regions by optimizing the global welfare by using Joint Maximization. The non-cooperative solution considers spillovers as well, but they are not internalized. The relevant externalities are the technology learning effects in the energy sector.


==Production structure==


REMIND uses a nested production function with constant elasticity of substitution (CES) to determine region?s gross domestic product (GDP) (see Figure 3 ). Inputs at the upper level of the production function include labor, capital, and final energy. We use the population at working age to determine labor. Final energy input to the upper production level forms a CES nest, which comprises energy for transportation and stationary energy coupled with a substitution elasticity of 0.3. In turn, these two energy types are, determined by the nested CES functions of more specific final energy carriers. REMIND assumes substitution elasticities between 1.5 and 3 for the lower levels of the CES nest. It assigns an efficiency parameter to each production factor in the various macroeconomic CES functions. Exogenous scenarios provide changes in the efficiencies of the individual production factors for each region.


The macro-economic budget constraint for each region ensures that  in each region and for every time step, the sum of GDP Yrt and imports of composite goods MGrt can be spent on consumption Crt, investments into the macroeconomic capital stock Irt (depreciation rate of 5%), energy system expenditures Ert and the export of composite goods MGrt. Energy system expenditures consist of investment costs, fuel costs, and operation and maintenance costs.


[[File:REMIND_production_structure.JPG]]


The balance of demand from the macro-economy and supply from the energy system delivers equilibrium prices at the final energy level.


[[File:REMIND production structure 2.png]]


Figure 3. Production structure of REMIND. Linear production functions describe the conversion of primary energy (lowest level) to final energy carriers. Nested CES structures describe the aggregation of final energy carriers for end-use.


==Trade==


REMIND considers the trade of coal, gas, oil, biomass, uranium, the composite good (aggregated output of the macro-economic system), and emissions permits (in the case of climate policy). It assumes that renewable energy sources (other than biomass) and secondary energy carriers are non-tradable across regions. As an exception, REMIND can consider bilateral trade in electricity between specific region pairs (e.g., Europe and North Africa / Middle East). According to energy statistics, trade in refined liquid fuels does take place in the real world, but to a smaller extent than crude oil. Since REMIND considers crude oil trade, the liquid fuel trade only has a small share and is attributed to crude oil trade. To be consistent with trade statistics, REMIND allocates the trade in petroleum products to crude oil trade.


For each good i a global trade balance equation ensures that markets are cleared:


[[File:REMIND trade 1.JPG]]
<references />
 
REMIND models regional trade via a common pool, with the exception of the bilateral electrity trade mentioned above. While each region is an open system - meaning that it can import more than it exports - the global system is closed. The combination of regional budget constraints and international trade balances ensures that the sum of regional consumption, investments, and energy-system expenditures cannot be greater than the global total output in each period. In line with the classical Heckscher-Ohlin and Ricardian models (Heckscher et al. 1991), trade between regions is induced by differences in factor endowments and technologies. REMIND represents the additional possibility of inter-temporal trade. This means that for each region, the value of exports must balance the value of imports within the time horizon of the model. This is ensured by the inter-temporal budget constraint, where ?ir is the present value price of good i.
 
[[File:REMIND trade 2.JPG]]
 
Therefore, discounting is implicit. Alternatively, this can be interpreted as capital trade or borrowing and lending. Inter-temporal trade and the capital mobility implied by trade in the composite good, cause mobile factor prices to equalize, thus providing the basis for an inter-temporal and inter-regional equilibrium. Since no capital market distortions are considered, the interest rates equalize across regions. Similarly, permit prices equalize across regions, unless their trade is restricted. By contrast, final energy prices and wages can differ across regions because these factors are immobile. Prices for traded primary energy carriers differ according to the transportation costs.
 
[[File:REMIND trade 3.JPG]]
 
Trade balances imply that the regional current accounts (and their counterparts - capital accounts) have a sum of zero at each point in time. In other words, regions with a current account surplus balance regions with a current account deficit. Budget constraints inter-temporally balance debts and assets that accrue through trade. This means that an export surplus qualifies the exporting region for an import surplus (of the same present value) in the future, thus also implying a loss of consumption for the current period. REMIND models trading of emissions permits in a similar way. In the presence of a global carbon market, the initial allocation of emissions rights is determined by a burden-sharing rule wherein permits can be freely traded among world regions. A permit-constraint equation ensures that an emissions certificate covers each unit of GHG emissions.
 
The trading of resources is subject to trade costs. In terms of consumable generic goods, the representative households in REMIND are indifferent to domestic and foreign goods as well as foreign goods from different origins. This can potentially lead to a strong specialization pattern.
 
The treatment of trade in REMIND depends on the solution concept (Nash vs. Negishi). The two approaches are in a dual relationship. The Negishi approach considers the trade balances of all goods explicitly and adjusts the welfare weights in order to guarantee that the intertemporal balance of payments of each region is settled. REMIND derives the prices of traded goods from the optimal solution in each iteration. The Nash approach adjusts goods prices until demand and supply of traded goods are equalized. In each iteration, the international prices are exogenous parameters for all regions. Furthermore, each region is subject to an intertemporal budget constraint, i.e. the intertemporal balance of payments has to be equal to zero.
 
Table 3. Characterization of the treatment of trade in the two alternative Negishi and Nash solution concepts.
 
[[File:REMIND trade 4.JPG]]

Latest revision as of 14:46, 12 June 2023

Model Documentation - REMIND-MAgPIE

Corresponding documentation
Previous versions
Model information
Model link
Institution Potsdam Institut für Klimafolgenforschung (PIK), Germany, https://www.pik-potsdam.de.
Solution concept General equilibrium (closed economy)MAgPIE: partial equilibrium model of the agricultural sector;
Solution method OptimizationMAgPIE: cost minimization;
Anticipation

Note: This pages describes the REMIND 1.7 model. It will be updated shortly to describe the most recent version of REMIND-MAgPIE.

Objective function

REMIND-MAgPIE models each region r as a representative household with a utility function Ur that depends upon per-capita consumption

<figure id="fig:REMIND-MAgPIE_3.2.1 1."> REMIND-MAgPIE equation 3.2.1 1.JPG </figure>

where C(r,t) is the consumption of region r at time t, and P(r,t) is the population in region r at time t. The calculation of utility is subject to discounting; 3% is assumed for the pure rate of time preference rho. The logarithmic relationship between per-capita consumption and regional utility implies an elasticity of marginal consumption of 1. Thus, in line with the Keynes-Ramsey rule, REMIND-MAgPIE yields an endogenous interest rate in real terms of 5–6% for an economic growth rate of 2–3%. This is in line with the interest rates typically observed on capital markets.

REMIND-MAgPIE can compute maximum regional utility (welfare) by two different solution concepts – the Negishi approach and the Nash approach [1]. In the Negishi approach, which computes a cooperative solution, the objective of the Joint Maximization Problem is the weighted sum of regional utilities, maximized subject to all other constraints:

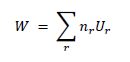
<figure id="fig:REMIND-MAgPIE_3.2.1 2."> REMIND-MAgPIE equation 3.2.1 2.JPG </figure>

An iterative algorithm adjusts the weights so as to equalize the intertemporal balance of payments of each region over the entire time horizon. This convergence criterion ensures that the Pareto-optimal solution of the model corresponds with the market equilibrium in the absence of non-internalized externalities. The algorithm is an inter-temporal extension of the original Negishi approach [2]; see also [3] for a discussion of the extension. Other models such as MERGE [4] and RICE50+ [5] use this algorithm in a similar way.

The Nash solution concept, by contrast, arrives at the Pareto solution not by Joint Maximization, but by maximizing the regional welfare subject to regional constraints and international prices that are taken as exogenous data for each region. The intertemporal balance of payments of each region has to equal zero and is one particular constraint imposed on each region. The equilibrium solution is found by iteratively adjusting the international prices until global demand and supply are balanced on each market. The choice of the solution concept is also important for the representation of trade, as discussed in Section the section on Trade.

In contrast to the Negishi approach, which solves for a co-operative Pareto solution, the Nash approach solves for a non-cooperative Pareto solution. The cooperative solution internalizes interregional spillovers between regions by optimizing the global welfare by using Joint Maximization. The non-cooperative solution considers spillovers as well, but they are not internalized. The relevant externalities are the technology learning effects in the energy sector.






  1. ↑ Leimbach M, Schultes A, Baumstark L, et al (2016) Solution algorithms of large‐scale Integrated Assessment models on climate change. Annals of Operations Research, doi:10.1007/s10479-016-2340-z
  2. ↑ Negishi T (1972) General equilibrium theory and international trade. North-Holland Publishing Company Amsterdam, London
  3. ↑ Manne AS, Rutherford TF (1994) International Trade in Oil, Gas and Carbon Emission Rights: An Intertemporal General Equilibrium Model. The Energy Journal Volume15:57–76
  4. ↑ Manne A, Mendelsohn R, Richels R (1995) MERGE: A model for evaluating regional and global effects of GHG reduction policies. Energy Policy 23:17–34. doi: 10.1016/0301-4215(95)90763-W
  5. ↑ Nordhaus WD, Yang Z (1996) A Regional Dynamic General-Equilibrium Model of Alternative Climate-Change Strategies. The American Economic Review 86:741–765
Retrieved from "https://www.iamcdocumentation.eu/index.php?title=Macro-economy_-_REMIND-MAgPIE&oldid=15936"